error msg
- Algebra
- Calculus
- Trigonometry
- Matrix
- Differential
- Integral
- Trigonometry
- Letters
Question
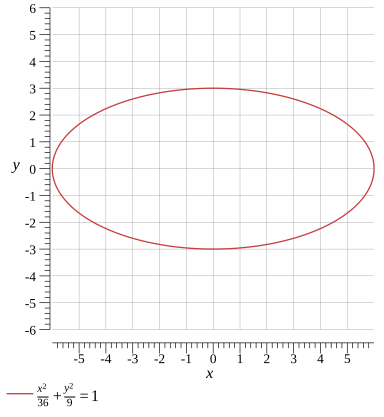
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Identify the conic
-
Find the center of the ellipse
-
Find the foci of the ellipse
-
Find the vertices of the ellipse
-
Find the eccentricity of the ellipse
More methods
Hide more
\left(0,0\right)
Rewrite in standard form
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Solution
\left(0,0\right)
Show Solutions
Solve the equation
-
\text{Solve for }x
-
\text{Solve for }y
\begin{align}&x=2\sqrt{9-y^{2}}\\&x=-2\sqrt{9-y^{2}}\end{align}
Evaluate
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Move the expression to the right-hand side and change its sign
\frac{x^{2}}{36}=1-\frac{y^{2}}{9}
Subtract the terms
More Steps
Evaluate
1-\frac{y^{2}}{9}
Reduce fractions to a common denominator
\frac{9}{9}-\frac{y^{2}}{9}
Write all numerators above the common denominator
\frac{9-y^{2}}{9}
\frac{x^{2}}{36}=\frac{9-y^{2}}{9}
\text{Multiply both sides of the equation by }36
\frac{x^{2}}{36}\times 36=\frac{9-y^{2}}{9}\times 36
Multiply the terms
x^{2}=\frac{\left(9-y^{2}\right)\times 36}{9}
Evaluate
x^{2}=36-4y^{2}
Take the root of both sides of the equation and remember to use both positive and negative roots
x=\pm \sqrt{36-4y^{2}}
Simplify the expression
More Steps
Evaluate
\sqrt{36-4y^{2}}
Factor the expression
\sqrt{4\left(9-y^{2}\right)}
The root of a product is equal to the product of the roots of each factor
\sqrt{4}\times \sqrt{9-y^{2}}
Evaluate the root
More Steps
Evaluate
\sqrt{4}
\text{Write the number in exponential form with the base of }2
\sqrt{2^{2}}
\text{Reduce the index of the radical and exponent with }2
2
2\sqrt{9-y^{2}}
x=\pm 2\sqrt{9-y^{2}}
Solution
\begin{align}&x=2\sqrt{9-y^{2}}\\&x=-2\sqrt{9-y^{2}}\end{align}
Show Solutions
Testing for symmetry
-
Testing for symmetry about the origin
-
Testing for symmetry about the x-axis
-
Testing for symmetry about the y-axis
\textrm{Symmetry with respect to the origin}
Evaluate
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
\text{To test if the graph of }\frac{x^{2}}{36}+\frac{y^{2}}{9}=1\text{ is symmetry with respect to the origin,substitute -x for x and -y for y}
\frac{\left(-x\right)^{2}}{36}+\frac{\left(-y\right)^{2}}{9}=1
Evaluate
More Steps
Evaluate
\frac{\left(-x\right)^{2}}{36}+\frac{\left(-y\right)^{2}}{9}
Rewrite the expression
\frac{x^{2}}{36}+\frac{y^{2}}{9}
Reduce fractions to a common denominator
\frac{x^{2}}{36}+\frac{y^{2}\times 4}{9\times 4}
Multiply the numbers
\frac{x^{2}}{36}+\frac{y^{2}\times 4}{36}
Write all numerators above the common denominator
\frac{x^{2}+y^{2}\times 4}{36}
Use the commutative property to reorder the terms
\frac{x^{2}+4y^{2}}{36}
\frac{x^{2}+4y^{2}}{36}=1
Solution
\textrm{Symmetry with respect to the origin}
Show Solutions
Find the first derivative
-
\text{Find the derivative with respect to }x
-
\text{Find the derivative with respect to }y
\frac{dy}{dx}=-\frac{x}{4y}
Calculate
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Take the derivative of both sides
\frac{d}{dx}\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)=\frac{d}{dx}\left(1\right)
Calculate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)
Use differentiation rules
\frac{d}{dx}\left(\frac{x^{2}}{36}\right)+\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Evaluate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{x^{2}}{36}\right)
Rewrite the expression
\frac{\frac{d}{dx}\left(x^{2}\right)}{36}
\text{Use }\frac{d}{dx} x^{n}=n x^{n-1}\text{ to find derivative}
\frac{2x}{36}
Calculate
\frac{x}{18}
\frac{x}{18}+\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Evaluate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Rewrite the expression
\frac{\frac{d}{dx}\left(y^{2}\right)}{9}
Evaluate the derivative
\frac{2y\frac{dy}{dx}}{9}
\frac{x}{18}+\frac{2y\frac{dy}{dx}}{9}
Calculate
\frac{x+4y\frac{dy}{dx}}{18}
\frac{x+4y\frac{dy}{dx}}{18}=\frac{d}{dx}\left(1\right)
Calculate the derivative
\frac{x+4y\frac{dy}{dx}}{18}=0
Simplify
x+4y\frac{dy}{dx}=0
Move the constant to the right side
4y\frac{dy}{dx}=0-x
Removing 0 doesn't change the value,so remove it from the expression
4y\frac{dy}{dx}=-x
Divide both sides
\frac{4y\frac{dy}{dx}}{4y}=\frac{-x}{4y}
Divide the numbers
\frac{dy}{dx}=\frac{-x}{4y}
Solution
\frac{dy}{dx}=-\frac{x}{4y}
Show Solutions
Find the second derivative
-
\text{Find the second derivative with respect to }x
-
\text{Find the second derivative with respect to }y
\frac{d^2y}{dx^2}=-\frac{4y^{2}+x^{2}}{16y^{3}}
Calculate
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Take the derivative of both sides
\frac{d}{dx}\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)=\frac{d}{dx}\left(1\right)
Calculate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)
Use differentiation rules
\frac{d}{dx}\left(\frac{x^{2}}{36}\right)+\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Evaluate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{x^{2}}{36}\right)
Rewrite the expression
\frac{\frac{d}{dx}\left(x^{2}\right)}{36}
\text{Use }\frac{d}{dx} x^{n}=n x^{n-1}\text{ to find derivative}
\frac{2x}{36}
Calculate
\frac{x}{18}
\frac{x}{18}+\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Evaluate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(\frac{y^{2}}{9}\right)
Rewrite the expression
\frac{\frac{d}{dx}\left(y^{2}\right)}{9}
Evaluate the derivative
\frac{2y\frac{dy}{dx}}{9}
\frac{x}{18}+\frac{2y\frac{dy}{dx}}{9}
Calculate
\frac{x+4y\frac{dy}{dx}}{18}
\frac{x+4y\frac{dy}{dx}}{18}=\frac{d}{dx}\left(1\right)
Calculate the derivative
\frac{x+4y\frac{dy}{dx}}{18}=0
Simplify
x+4y\frac{dy}{dx}=0
Move the constant to the right side
4y\frac{dy}{dx}=0-x
Removing 0 doesn't change the value,so remove it from the expression
4y\frac{dy}{dx}=-x
Divide both sides
\frac{4y\frac{dy}{dx}}{4y}=\frac{-x}{4y}
Divide the numbers
\frac{dy}{dx}=\frac{-x}{4y}
\text{Use }\frac{-a}{b}=\frac{a}{-b}=-\frac{a}{b}\text{ to rewrite the fraction}
\frac{dy}{dx}=-\frac{x}{4y}
Take the derivative of both sides
\frac{d}{dx}\left(\frac{dy}{dx}\right)=\frac{d}{dx}\left(-\frac{x}{4y}\right)
Calculate the derivative
\frac{d^2y}{dx^2}=\frac{d}{dx}\left(-\frac{x}{4y}\right)
Use differentiation rules
\frac{d^2y}{dx^2}=-\frac{\frac{d}{dx}\left(x\right)\times 4y-x\times \frac{d}{dx}\left(4y\right)}{\left(4y\right)^{2}}
\text{Use }\frac{d}{dx} x^{n}=n x^{n-1}\text{ to find derivative}
\frac{d^2y}{dx^2}=-\frac{1\times 4y-x\times \frac{d}{dx}\left(4y\right)}{\left(4y\right)^{2}}
Calculate the derivative
More Steps
Evaluate
\frac{d}{dx}\left(4y\right)
Simplify
4\times \frac{d}{dx}\left(y\right)
Calculate
4\frac{dy}{dx}
\frac{d^2y}{dx^2}=-\frac{1\times 4y-x\times 4\frac{dy}{dx}}{\left(4y\right)^{2}}
Any expression multiplied by 1 remains the same
\frac{d^2y}{dx^2}=-\frac{4y-x\times 4\frac{dy}{dx}}{\left(4y\right)^{2}}
Use the commutative property to reorder the terms
\frac{d^2y}{dx^2}=-\frac{4y-4x\frac{dy}{dx}}{\left(4y\right)^{2}}
Calculate
More Steps
Evaluate
\left(4y\right)^{2}
Evaluate the power
4^{2}y^{2}
Evaluate the power
16y^{2}
\frac{d^2y}{dx^2}=-\frac{4y-4x\frac{dy}{dx}}{16y^{2}}
Calculate
\frac{d^2y}{dx^2}=-\frac{y-x\frac{dy}{dx}}{4y^{2}}
\text{Use equation }\frac{dy}{dx}=-\frac{x}{4y}\text{ to substitute}
\frac{d^2y}{dx^2}=-\frac{y-x\left(-\frac{x}{4y}\right)}{4y^{2}}
Solution
More Steps
Calculate
-\frac{y-x\left(-\frac{x}{4y}\right)}{4y^{2}}
Multiply the terms
More Steps
Evaluate
x\left(-\frac{x}{4y}\right)
Multiplying or dividing an odd number of negative terms equals a negative
-x\times \frac{x}{4y}
Multiply the terms
-\frac{x\times x}{4y}
Multiply the terms
-\frac{x^{2}}{4y}
-\frac{y-\left(-\frac{x^{2}}{4y}\right)}{4y^{2}}
Subtract the terms
More Steps
Simplify
y-\left(-\frac{x^{2}}{4y}\right)
If a negative sign or a subtraction symbol appears outside parentheses, remove the parentheses and change the sign of every term within the parentheses
y+\frac{x^{2}}{4y}
Reduce fractions to a common denominator
\frac{y\times 4y}{4y}+\frac{x^{2}}{4y}
Write all numerators above the common denominator
\frac{y\times 4y+x^{2}}{4y}
Multiply the terms
\frac{4y^{2}+x^{2}}{4y}
-\frac{\frac{4y^{2}+x^{2}}{4y}}{4y^{2}}
Divide the terms
More Steps
Evaluate
\frac{\frac{4y^{2}+x^{2}}{4y}}{4y^{2}}
Multiply by the reciprocal
\frac{4y^{2}+x^{2}}{4y}\times \frac{1}{4y^{2}}
Multiply the terms
\frac{4y^{2}+x^{2}}{4y\times 4y^{2}}
Multiply the terms
\frac{4y^{2}+x^{2}}{16y^{3}}
-\frac{4y^{2}+x^{2}}{16y^{3}}
\frac{d^2y}{dx^2}=-\frac{4y^{2}+x^{2}}{16y^{3}}
Show Solutions
Rewrite the equation
\begin{align}&r=\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}\\&r=-\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}\end{align}
Evaluate
\frac{x^{2}}{36}+\frac{y^{2}}{9}=1
Multiply both sides of the equation by LCD
\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)\times 36=1\times 36
Simplify the equation
More Steps
Evaluate
\left(\frac{x^{2}}{36}+\frac{y^{2}}{9}\right)\times 36
Apply the distributive property
\frac{x^{2}}{36}\times 36+\frac{y^{2}}{9}\times 36
Simplify
x^{2}+y^{2}\times 4
Use the commutative property to reorder the terms
x^{2}+4y^{2}
x^{2}+4y^{2}=1\times 36
Any expression multiplied by 1 remains the same
x^{2}+4y^{2}=36
\text{To convert the equation to polar coordinates,substitute }x\text{ for }r\cos\left(\theta \right)\text{ and }y\text{ for }r\sin\left(\theta \right)
\left(\cos\left(\theta \right)\times r\right)^{2}+4\left(\sin\left(\theta \right)\times r\right)^{2}=36
Factor the expression
\left(\cos^{2}\left(\theta \right)+4\sin^{2}\left(\theta \right)\right)r^{2}=36
Simplify the expression
\left(-3\cos^{2}\left(\theta \right)+4\right)r^{2}=36
Divide the terms
r^{2}=\frac{36}{-3\cos^{2}\left(\theta \right)+4}
Simplify the expression
r^{2}=\frac{36}{1+3\sin^{2}\left(\theta \right)}
Evaluate the power
r=\pm \sqrt{\frac{36}{1+3\sin^{2}\left(\theta \right)}}
Simplify the expression
More Steps
Evaluate
\sqrt{\frac{36}{1+3\sin^{2}\left(\theta \right)}}
To take a root of a fraction,take the root of the numerator and denominator separately
\frac{\sqrt{36}}{\sqrt{1+3\sin^{2}\left(\theta \right)}}
Simplify the radical expression
More Steps
Evaluate
\sqrt{36}
\text{Write the number in exponential form with the base of }6
\sqrt{6^{2}}
\text{Reduce the index of the radical and exponent with }2
6
\frac{6}{\sqrt{1+3\sin^{2}\left(\theta \right)}}
Multiply by the Conjugate
\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{\sqrt{1+3\sin^{2}\left(\theta \right)}\times \sqrt{1+3\sin^{2}\left(\theta \right)}}
Calculate
\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}
r=\pm \frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}
Solution
\begin{align}&r=\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}\\&r=-\frac{6\sqrt{1+3\sin^{2}\left(\theta \right)}}{1+3\sin^{2}\left(\theta \right)}\end{align}
Show Solutions
Graph