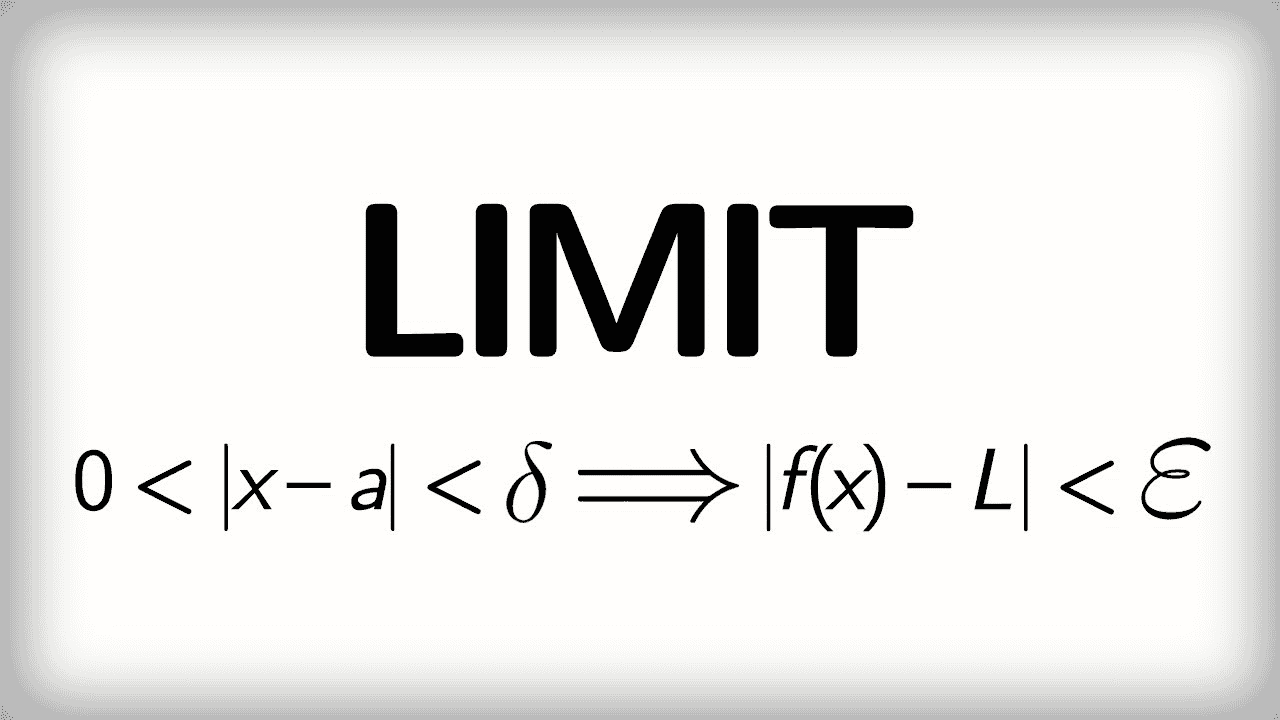What Is a Derivative?

Have you ever considered how altering car speed impacts travel distance or raising production at a company affects profit or costs? These inquiries involve change; mathematics' derivative concept offers powerful tools for understanding more fully this phenomenon.

The History and Development of Derivatives

Derivatives, as we know them today, have a rich history that can be traced back to real-world problem-solving techniques. Over time, they have evolved into the formal mathematical instruments we use in calculus.

Newton and Leibniz

Sir Isaac Newton and Gottfried Wilhelm Leibniz developed calculus independently during the 17th century. Newton used an approach that took into account physical concepts like velocity and acceleration as rates of change; such rates of change became known in Newton's studies of planetary motion as "fluxions". Leibniz formalized calculus mathematically through his modern notation $\frac{dy}{dx}$ , allowing small changes between variables with greater precision and control than what Newton offered when approaching derivatives from physical perspectives. Both laid the groundwork for later developments of derivatives! No matter who founded calculus first!

Over time, derivatives have slowly transitioned from real-world problem-solving strategies into formal mathematical tools.

Modern Foundations

With rigorous formalization, Augustin-Louis Cauchy and Karl Weierstrass revolutionized calculus during the 19th century. Cauchy's precise epsilon-delta definition of limits replaced intuitive ideas of infinitesimals to provide a mathematical basis for derivations; Weierstrass further improved this work by eliminating all ambiguity from calculus as it adhered to an exact and rigorous mathematical model; their work ultimately transformed derivatives into an easily applicable formal tool applicable across many fields and problems, making calculus one of classical mathematics's most trusted systems.

Applications Today

Since the 20th and 21st centuries, derivatives have played an essential part in computational sciences applications like machine learning and optimization, which utilize gradient calculations in order to reduce errors. Still, beyond computational applications, derivatives also play important roles in engineering simulations, economics, and data analysis, as well as more general applications, which demonstrate their versatility and relevance.

The Meaning of Derivatives in Calculus  

Derivatives are at the core of calculus and provide a precise mathematical tool for measuring change. Derivatives allow us to understand how minor adjustments in one variable may have an effect on another variable, whether analyzing physical motion, economic trends, or machine learning models; derivatives provide a means to quantify variability and change.

Basic Concept (Graphical Representation)  

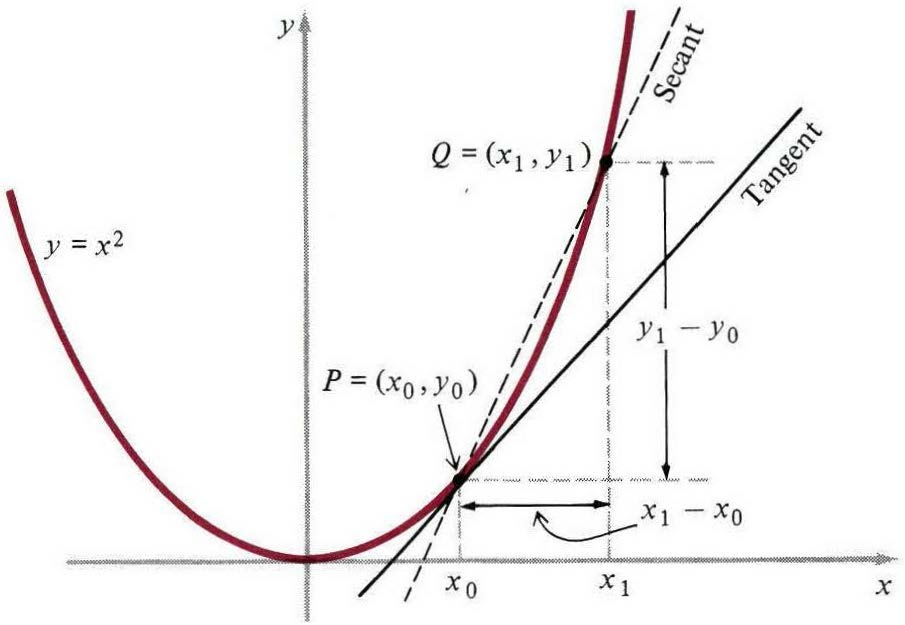
Geometrically speaking, a derivative at any point on a curve represents its slope at that location on its tangent line; more simply put, this measure captures the "steepness" of its curvature at that particular position. If analyzing graphs of vehicle distance against time over time, derivative at certain times would reveal its instantaneous speed.

A tangent line differs from secant lines in that it only touches a curve at one location without crossing it nearby, providing insight into its behavior at that spot. Derivative values provide visual and spatial interpretations, enabling us to better analyze data changes over time.

Symbolic Representation

Mathematically, derivatives (denoted by ( $f(x)$or$\frac{dy}{dx}$) measure minute changes. If y is position and x is time, $\frac{dy}{dx}$ equals velocity. Derivatives provide both symbolic and graphical tools, making them invaluable tools for both theoretical and practical analysis.

Fascinating Mathematical Applications of Derivatives

Derivatives are more than mere mathematical concepts; they have practical uses across numerous fields. Their application provides crucial insight into how functions behave, helping us efficiently address real-world issues. Their practical applications make derivatives an indispensable resource in mathematicians' and scientists' hands.

Slope of the Tangent Line

Definition of the Tangent Line

Tangent lines at any particular point along a curve are straight lines that connect directly with its curve, touching without intersecting nearby, to indicate its immediate direction - its "instantaneous behavior."

Calculating the Tangent Line's Slope

The slope of this tangent line may be determined using the derivative of a function at any specific point; for instance, functions with equation $y=f(x)$ have their zero slope determined using their derivative at that location  $x=x_0$ is equivalent to its derivative being called out as such at point zero in its equation; similarly for functions like those defined as having equation$f(x)=x^2$, its derivative at 1 would be known as $f^{\prime }(1)=2\times 1=2$ which provides its slope.

Rate of Instantaneous Change

What is the Rate of Change?

The rate of change measures how one quantity alters in response to another; its derivative precisely expresses this rate at any moment in time. Assume, for instance, that car's position can be represented using time as its variable of interest and derivative $\frac{ds}{dt}$ provides instantaneous speed at that instant in time.

Applications of the Rate of Change

This concept can be found throughout physics (velocity and acceleration), biology (population growth), and economics (profit changes). When applied to profit functions P(q), in which Q is quantity produced and P' (q) represents marginal profit per unit produced, derivatives provide predictive solutions and support decision making processes in dynamic environments.

Optimizing Functions: Maximization and Minimization

One key application of derivatives is optimization - finding maximum or minimum values of functions. By searching where the first derivative of an equation equals zero, we locate critical points that may represent potential maxima or minima of that function and later analyze these with regard to its second derivative to understand exactly its behavior - tools heavily applied across engineering, business, and technology in order to increase performance and efficiency.

Analyzing a Function's Behavior

Derivatives provide more than just value optimization - they also reveal insights into a function's behavior: 1. Growth and Decay:

When the value $f^{\prime }(x)>0$, growth occurs, while when it drops below 0, decay occurs, allowing us to understand trends over specific intervals.

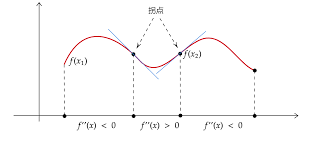
2. Concavity: The second derivative $f"(x)$ measures whether a curve is concave up (if its second derivative exceeds zero) or concave down ($f"(x)<0$). This tool helps predict whether changes are speeding up or slowing down over time.

3. Finding Extrema: Exploring global maxima and minima within restricted intervals can yield valuable insight for decision-making problems like cutting costs or expanding profits.

From predicting trajectory trajectories in physics to examining marginal changes in economics, derivatives serve as invaluable tools. Their versatility enables us to comprehend relationships, optimize systems, and make informed decisions in our ever-evolving world.

Derivatives from First Principles  

An effective understanding of derivatives requires going back over their basic definition, with their definition as limits as the focal point in mind. Examining first principles further and studying mathematical studies of change to develop a further appreciation of them are also keys to providing an in-depth knowledge base about them.

Definition Formula  

Limit Definition  

At its heart, a derivative of any function  ($f(x)$in our example) at any specific point $x_0$ can be understood as being limited by average rate of change as the interval nears zero - its formula reads thusly:

$f^{\prime }(x_0)={\lim }_{h\to 0}\frac{f(x_0+h)-f(x_0)}{h}$

The numerator $f(x_0+h)-f(x_0)$ measures changes in $y$ values; whilst denominator, which in this instance measures changes to $x$ values. At any moment when these measurements cross each other $h\to 0$ , an instantaneous rate of change exists when the secant line becomes a tangent line and vice versa.

This definition provides the basis for understanding derivatives--it encapsulates their essence of being measures that monitor how one quantity changes with regards to changes in another quantity, instantaneously and precisely.

Formula Derivation  

Let us consider an equation with limit definition $f(x)=x^3$and use its derivative as its limit definition to compute its derivative at any point x_0:

$f^{\prime }(x_0)={\lim }_{h\to 0}\frac{(x_0+h{)}^3-x_0^3}{h}$

Expanding and simplifying:  

$f^{\prime }(x_0)={\lim }_{h\to 0}\frac{x_0^3+3h{x}_0^2+3x_0{h}^2+h^3-x_0^3}{h}={\lim }_{h\to 0}\frac{3h{x}_0^2+3x_0{h}^2+h^3}{h}$

Factoring out $h$:  

$f^{\prime }(x_0)={\lim }_{h\to 0}(3x_0^2+3x_{0}h+h^2)=3x_0^2$

Thus, the derivative of $f(x)=x^3$ is $f^{\prime }(x)=3x^2$. This approach illustrates how derivatives connect the abstract idea of a limit with concrete calculations.  

Three Standard Derivative Formulas  

The Power Rule

Power laws simplify the differentiation of any power function of the form $f(x)=x^n$:

$f^{\prime }(x)=n\cdot x^{n-1}$

This rule can be used universally across the real numbers $n$, from integers and negative numbers through integers, fractions, and negative numbers. As an illustration of its application: for the derivative of $f(x)=x^3$, we get $f^{\prime }(x)=3x^2$, Likewise with other real number derivatives $f(x)=\frac{1}{x}$ results in $f^{\prime }(x)=-\frac{1}{x^2}$.

Derivative of Logarithmic and Exponential Functions

Logarithmic Functions  

For natural logarithm functions such as $f(x)=\ln (x)$, the derivative can be expressed as follows.

$f^{\prime }(x)=\frac{1}{x},x>0$

This formula derives from logarithms and illustrates their slowening rate as values increase; an example would be: $f^{\prime }(10)=1/10$. As higher values grow, their growth becomes slower.

Exponential Functions  

Exponential functions, like that $f(x)=e^x$, have unique derivatives in that their value corresponds with that of their function itself.

$f^{\prime }(x)=e^x$

This property of $e^x$ makes it one of the fundamental functions in calculus and application, particularly among exponential functions whose exponent exceeds zero; general exponential functions with exponents greater than zero can have their derivative expressed via:

General exponential functions $f(x)=a^x$ with exponents greater than zero can have their derivative expressed using:

$f^{\prime }(x)=a^x\ln (a)$

For example, if $f(x)=2^x$, then $f^{\prime }(x)=2^x\ln (2)$. This formula allows us to calculate derivatives for all forms of exponential growth models easily.

Derivatives of Trigonometric Functions

Basic Trigonometric Functions  

Sine and cosine functions are two fundamental trigonometric functions that can be expressed using their derivatives:

$\frac{d}{dx}[\sin (x)]=\cos (x),\frac{d}{dx}[\cos (x)]=-\sin (x)$

These relationships, derived from the unit circle, offer an effective means for studying oscillatory motion such as waves or periodic events.

Example 1: Given$y=\sin (x)$, its derivative gives us an instantaneous rate of change at any point along the curve $y^{\prime }=\cos (x)$

Inverse Trigonometric Functions  

Calculus makes heavy use of derivatives of trigonometric functions with an inverse, such as these:

$\frac{d}{dx}[\arcsin (x)]=\frac{1}{\sqrt{1-x^2}},\frac{d}{dx}[\arctan (x)]=\frac{1}{1+x^2}$

Trigonometric functions derived through implicit differentiation form an indispensable resource when solving integrals and equations with angles, integrals being treated like equations with angles as variables. Standard derivative formulae form the backbone for understanding more advanced applications of derivatives within calculus.

Fundamental Derivative Rules  

Fundamental derivative rules provide an efficient solution to multiple function differentiation by quickly computing derivatives without resorting to the direct limit definition. As they're great tools for dealing with real-world issues related to rates of change, such as motion optimization or growth trends - fundamental derivative rules prove indispensable in solving real problems such as those presented here.

The Power Rule

The power rule applies to functions with a form such as$f(x)=x^n$. Their derivative can be expressed using:  

$f^{\prime }(x)=n\cdot x^{n-1}$

This rule is both simple and versatile; for instance, its derivative for an equation like $f(x)=x^3$ is $f^{\prime }(x)=3x^2$; conversely for an expression like $f(x)=\sqrt{x}$ (i.e., $x^{1/2}$) the derivative would be: "$f^{\prime }(x)=\frac{1}{2}{x}^{-1/2}$" Furthermore, The power rule can accommodate positive as well as negative exponents.

The Sum/Difference Rule

According to the Sum/Difference Rule, when two functions are combined or subtracted from each other, their derivative will equal either their sum or difference of individual derivatives - mathematically speaking:

$\frac{d}{dx}[u(x)+v(x)]=u^{\prime }(x)+v^{\prime }(x)$

$\frac{d}{dx}[u(x)-v(x)]=u^{\prime }(x)-v^{\prime }(x)$

Example 1. If $f(x)=x^3+6x$, then its derivative is:$f^{\prime }(x)=\frac{d}{dx}[x^3]+\frac{d}{dx}[6x]=3x^2+6$ This rule simplifies differentiation by breaking complex functions into manageable pieces and improving accuracy and speed.

Example 2: If $g(x)=x^3-x$, then: $g^{\prime }(x)=3x^2-1$ This formula makes differentiation simpler by breaking complex functions down into manageable chunks that make differentiation simpler to perform.

The Product Rule

The Product Rule involves the combination of two functions, such as $u(x)$and $v(x)$, to produce their derivative, which in this application could be represented as:

$\frac{d}{dx}[u(x)\cdot v(x)]=u^{\prime }(x)v(x)+u(x)v^{\prime }(x)$

For example, if $f(x)=x^2\cdot \sin (x)$, then:  

$f^{\prime }(x)=\frac{d}{dx}[x^2]\cdot \sin (x)+x^2\cdot \frac{d}{dx}[\sin (x)]$

$f^{\prime }(x)=2x\cdot \sin (x)+x^2\cdot \cos (x)$

The product rule offers an effective means of managing functions where terms must be multiplied together - which is often required in scientific and engineering applications.

The Quotient Rule.

The quotient rule can be applied when one function is divided by another function, for instance, $f(x)=\frac{u(x)}{v(x)}$. It follows from this that its formula would be:

$\frac{d}{dx}\left[\frac{u(x)}{v(x)}\right]=\frac{u^{\prime }(x)v(x)-u(x)v^{\prime }(x)}{[v(x){]}^2}$

For example, if $f(x)=\frac{x^2}{\sin (x)}$, then:  

$f^{\prime }(x)=\frac{\frac{d}{dx}[x^2]\cdot \sin (x)-x^2\cdot \frac{d}{dx}[\sin (x)]}{[\sin (x){]}^2}$

$f^{\prime }(x)=\frac{2x\cdot \sin (x)-x^2\cdot \cos (x)}{[\sin (x){]}^2}$

The rule is essential when working with rational functions.

The Constant Multiple Rule   

The Constant Multiple Rule states that when multiplying any function by a constant $c$, its derivative will simply be equal to multiplying this constant multiply by its derivative of that function:

$\frac{d}{dx}[c\cdot u(x)]=c\cdot u^{\prime }(x)$

For example, if $f(x)=8x^2$, then:  

$f^{\prime }(x)=8\cdot \frac{d}{dx}[x^2]=8\cdot 2x=16x$

This rule works well when coupled with other rules for diverse functions and applications.

These basic rules of differentiation analysis provide powerful tools that enable efficient and precise tackling of virtually every function efficiently and precisely.

Advanced Derivative Rules  

Complex functions call for more advanced rules of derivatives besides fundamental ones; when dealing with complex functions, more advanced rules like chain rule, implicit differentiation, parametric derivatives, higher order derivatives partial derivatives, logarithmic differentiation, as well as logarithmic differentiation may all require using derivatives for extrema determination using logarithms as they provide robust solutions to increasingly sophisticated issues. Derivatives provide this power and utility, enabling us to address increasingly sophisticated challenges when using them.

Derivatives of Composite Functions (Chain Rule)

Definition of Composite Functions  

Composite functions are formed when one function is applied to another function's result; typically, they take the form $f(g(x))$, with $g(x)$as the inner function and $u=g(x)$ serving as its outer counterpart.

Rule Definition  

To determine the derivative of a compound function$f(g(x))$, using the chain rule requires multiplying both derivatives of its outer function $f$, evaluated at inner function $g(x)$, multiplied with its derivative from within it:

$\frac{d}{dx}[f(g(x))]=f^{\prime }(g(x))\cdot g^{\prime }(x)$

Formula  

Let’s consider $f(x)=(2x+1{)}^3$.  

Here, the outer function is $f(u)=u^3$ and the inner function is $g(x)=2x+1$. Using the chain rule:

$f^{\prime }(x)=3[2x+1{]}^2\cdot 2=6(2x+1{)}^2$

This rule proves invaluable in fields like physics and machine learning, where nestled functions are prevalent.

Derivatives of Implicit Functions

Definition of Implicit Functions  

An implicit function does not explicitly relate one variable to another variable but is instead defined by relationships among variables (for instance ( $F(x,y)=0$). An example would be: $x^2+y^2=1$ which describes a circle wherein $y$ ) depends upon $x$ even though these dependencies might not be explicit.

Rule Definition  

To differentiate implicit functions, we employ implicit differentiation by taking derivatives of both sides with respect to $x$, treating y as an output from$x$ ($y(x)$), and applying chain rule wherever needed.

Formula  

Consider $x^2+y^2=1$. Taking the derivative of both sides of $x$, we get:

$2x+2y\frac{dy}{dx}=0$

Solving for $\frac{dy}{dx}$:  

$\frac{dy}{dx}=-\frac{x}{y}$

Implicit differentiation is essential when solving problems where variables depend on one another, such as finding the tangents to curves or analyzing relationships in both physics and economics.

Parametric Derivatives  

Definition of Parametric Functions  

Parametric functions describe relationships between two variables using a third variable called a parameter, for instance, when used for motion analysis or trajectory mapping. An example would be where two parametric functions $x(t)$,$y(t)$ define coordinates of a curve as a function of $t$, often used for motion or trajectory analysis.

Rule Definition  

To find the derivative $\frac{dy}{dx}$, we first calculate $\frac{dx}{dt}$ and $\frac{dy}{dt}$, then use the formula:

$\frac{dy}{dx}=\frac{\frac{dy}{dt}}{\frac{dx}{dt}}$

Formula  

Consider $x(t)=t^2$ and $y(t)=t^3$.  

$\frac{dx}{dt}=2t,\frac{dy}{dt}=3t^2$

Then:  

$\frac{dy}{dx}=\frac{3t^2}{2t}=\frac{3t}{2}$

This approach can be especially helpful in the physical sciences for studying projectile motion or parametric curves.

Higher-Order Derivatives

Definition of Higher-Order Derivatives 

Higher-order derivatives can be produced by multiplying the derivatives of a function. The first derivative$f^{\prime }(x)$represents the rate of change, while the second derivative$f"(x)$describes the change in the rate of change, etc. Such derivatives have many uses in mechanics (for instance, acceleration is often considered the second derivative of position).

Rules  

Higher-order derivatives follow the same rules as first-order derivatives but must be applied iteratively.

Formula  

Consider $f(x)=x^3$:  

$f^{\prime }(x)=3x^2,f^{\prime \prime }(x)=6x,f^{\prime \prime \prime }(x)=6$

Applications include curve analysis, in which the function $f"(x)$ can be used to detect concavity and identify inflection points.

Partial Derivatives

Definition of Partial Derivatives  

Partial derivatives are useful when working with functions with multiple variables, like $f(x,y)$. They measure rate of change with respect to one variable while keeping all others constant.

Rule Definition  

To compute the partial derivative of $f(x,y)$ with respect to $x$, denoted by $\frac{\partial f}{\partial x}$, treat $y$ as an independent constant:

$\frac{\partial f}{\partial x}={\lim }_{\Delta x\to 0}\frac{f(x+\Delta x,y)-f(x,y)}{\Delta x}$

Formula  

For $f(x,y)=x^{2}y+y^3$:  

$\frac{\partial f}{\partial x}=2xy,\frac{\partial f}{\partial y}=x^2+3y^2$

Partial derivatives play an essential role in optimizing physics and multivariable systems such as thermodynamics.  

Using Logarithmic Differentiation  

Logarithmic differentiation makes the differentiation of functions with variable exponents easier.

Consider $f(x)=x^x$. Taking the natural logarithm:  

$\ln (f(x))=\ln (x^x)=x\ln (x)$

Differentiating both sides:  

$\frac{1}{f(x)}\cdot f^{\prime }(x)=\ln (x)+1$

Thus:  

$f^{\prime }(x)=x^x(\ln (x)+1)$

Logarithmic differentiation can be especially useful when working with complex products and exponential expressions.

Maximum and Minimum Values Using Derivatives

First Derivative Test

The First Derivative Test identifies critical points by solving for $f^{\prime }(x)=0$.

A critical point can be defined as either:- A local maximum when $f^{\prime }(x)$ changes from positive to negative or as an alternative minimum when changes go from negative to positive.

Example: For $f(x)=x^3-3x^2+4x$,  

$f^{\prime }(x)=3x^2-6x+4$  

Setting $f^{\prime }(x)=0$ and analyzing intervals can identify extrema.  

Second Derivative Test

The Second Derivative Test uses the function f" (x). If f" (x) > 0, this indicates a local minimum while, conversely, if f" (x) is lower than zero, this indicates an alternative local maximum condition.

Hessian matrix analysis (second partial derivatives) can be used to quickly find extrema in multivariable functions and optimization problems, providing valuable methods.

Applications of Derivatives in Economics

Marginal Cost and Marginal Benefit  

Derivatives have long been used in mathematics and physics; now, they play an essential part in economics as well. Economists use derivatives to quantify various relationships and rates of change relating to marginal cost, marginal benefit analysis, and elastic analysis, which are key pillars of decision-making support.

Marginal Cost  

Marginal changes or additions, while derivative calculations provide marginal cost and benefit calculations - two key concepts in production processes and decision-making decisions.

$MC=\frac{dC}{dq}$

For example, if $C(q)=5q^2+3q+10$, the marginal cost is:  

$MC=\frac{dC}{dq}=10q+3$

This means the cost to produce one additional unit increases linearly with $q$.

Marginal costs allow firms to determine how much production to undertake by comparing it against marginal revenue.

Marginal Benefit  

Marginal Benefit is defined as any additional utility realized from increasing consumption by one unit of any good, calculated against the total benefits function $B(q)$. When written as an equation based on total benefits function B(q), the marginal benefit equation may look something like this:

$MB=\frac{dB}{dq}$

An optimal production or consumption scenario can be defined when marginal cost equals marginal benefit ($MC=MB$), signaling equilibrium and increased efficiency.

Elasticity Analysis  

Elasticity in economics refers to how reactive one variable is to changes in another variable; derivative analysis plays an integral part in measuring it - particularly price elasticity of demand.

Price Elasticity of Demand  

Price Elasticity of Demand measures the degree to which the quantity desired responds to changes in price; it is defined as:

$E_p=\frac{\%\text{change in quantity demanded}}{\%\text{change in price}}=\frac{\frac{dQ}{dP}\cdot P}{Q}$

Here, (P ) refers to price; Q represents quantity desired, and $\frac{dQ}{dP}$ is its derivative with respect to price.

Example  

Suppose the demand function is $Q=100-2P$. Then:  

$\frac{dQ}{dP}=-2$

At $P=20$, the quantity demanded is $Q=100-2(20)=60$. The price elasticity is:  

$E_p=\frac{-2\cdot 20}{60}=-\frac{2}{3}$

This indicates that demand in this range is inelastic (less sensitive to price changes) than normal.

Understanding elastic demand helps businesses develop pricing strategies to optimize revenue. For instance, when E_p is above one, elastic demand exists, and decreasing prices would lead to greater returns.

If E_p<1, demand is inelastic, and therefore, increasing prices would result in more significant revenue generation.

Economic analysts use derivatives to understand marginal changes and elasticities so they can make more informed decisions regarding production, pricing, and resource allocation. Derivatives play an integral part in economic modeling and strategy creation.

This comprehensive guide explores the principles and applications of derivatives, from their role in solving complex mathematical problems to their historical development. Beginning with basic concepts such as tangent lines and rates of change, as well as advanced techniques like optimization, implicit differentiation and partial derivatives - this article makes derivatives accessible and practical for both mathematical theory and real world applications. From their roots with Newton and Leibniz to modern applications in physics, engineering, economics, and data science, derivatives have long been utilized as powerful tools for understanding and optimizing systems. From motion analysis to increasing profits - derivatives can play a pivotal role here! With this guide you'll have all the knowledge to leverage their full power!

reference：

https://en.wikipedia.org/wiki/Derivative

https://www.britannica.com/science/derivative-mathematics

https://www.khanacademy.org/math/differential-calculus/dc-diff-intro