What is calculus?
An introduction to the definition, history and application of calculus and supplement knowledge about its two main branches and related theorems and topics.
Alice Brooks
Dec 20, 2024 11 mins read
Calculus is the branch of mathematics that investigates rates of change. Imagine you're driving your car along the highway. Suddenly the traffic light turns green—how does your speed increase as soon as it begins moving towards its intersection, and how is acceleration calculated? These examples occur throughout daily life as our world constantly shifts and transforms; in this article we will look into their formation further. What is calculus? What is the rate of change?
Definition
Basic Definition
Calculus is fundamentally about understanding how things change and provides tools for quantifying changes, both linear and nonlinear. It explores function behaviors as they change with instantaneous effects as well as cumulative ones.
Two main branches
Calculus can be divided into two main branches, differential calculus and integral calculus. Differential calculus utilizes derivatives—rates of change that represent rates of change—as its focal point; it helps people better comprehend curve slopes, optimization problems, and motion, while integral calculus deals primarily with integrals to accumulate and analyze area under curves as a way of finding total quantities such as areas, volumes, or other cumulative measures. Both branches intertwine seamlessly, utilizing limits as the cornerstone concept in solving real-world issues, from speed calculations to finding areas under curves.
Etymology
Calculus has an intriguing origin in antiquity; its name derives from the Latin word for small stone (calculus). Ancient civilizations relied upon small stones or pebbles as counting tools for early arithmetic calculations—an early primitive form of counting that enabled merchants, traders, and scholars to perform basic mathematics operations like addition and subtraction. Over time calculus became associated with more sophisticated principles and systems of mathematical computation. By the Middle Ages, "calculus" became synonymous with any method or system for formal calculation. Isaac Newton and Gottfried Wilhelm Leibniz first pioneered our current mathematical framework during the 17th century; by then "calculus" had come to symbolize rigorous systematic processes used for studying rates of change and accumulation—reflecting its historical and methodological importance.
History
Here, we will offer a brief introduction and history review of calculus' invention.
The Precursors of Calculus
Calculus was not born overnight but rather came to existence through centuries of mathematical innovation and thought. Archimedes of ancient Greek mathematic fame developed the idea of "indivisibles," an early attempt to comprehend areas and volumes by decomposing their measurements into infinitely small quantities—an early precursor of modern infinitesimals. He utilized these ideas to calculate areas and volumes of circles and parabolas as well as volumes of spheres and cylinders. Liu Hui of ancient China utilized exhaustion—approximating areas by inscribing increasingly smaller polygons within them to approximate them—before integral calculus emerged by summarizing all their areas, thereby bridging between discrete and continuous quantities.
The Creation of Calculus
Isaac Newton and Gottfried Wilhelm Leibniz independently invented calculus during the late 17th century, marking a historic turning point in mathematical development. Newton made significant advances to science through his studies of motion and forces. He introduced the derivative to describe instantaneous rate changes—something integral for understanding his laws of motion and universal gravitation. Leibniz approached calculus from an academic viewpoint, using integral notation and systematic methods of calculation to establish calculus as a mathematical discipline. His notation for differentials greatly impacted modern representations and teaching of calculus; their combined efforts laid a unified mathematical framework capable of solving problems across geometry, physics, and beyond; however, their contributions gave rise to an ongoing controversy regarding which of them invented calculus first.
Basic Concepts of Differential Calculus
Limits
Limits form the core of calculus by showing us what happens as input approaches a certain value, providing insight into sudden, unanticipated, or unpredictable behavior from functions, like reaching infinity or sudden shifts. Below are examples of common limits:
- \(\lim_{{x \to \infty}} \frac{1}{x} = 0\)
- \(\lim_{{x \to 0}} \sin(x) = 0\)
- \(\lim_{{x \to 1}} (x^2 - 1) = 0\)
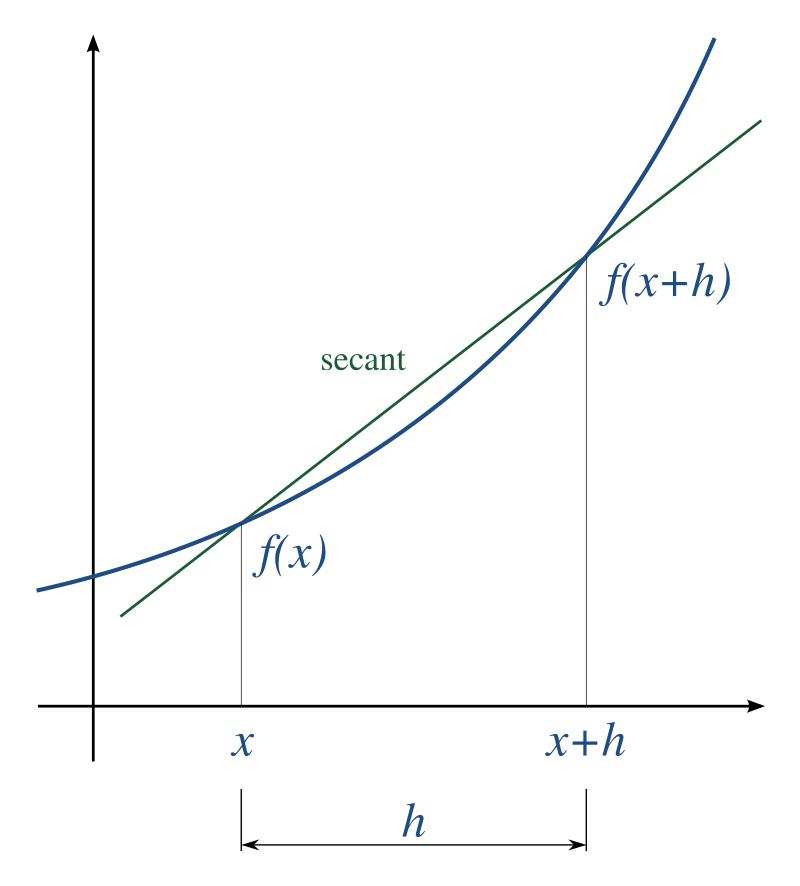
Derivatives
Definition
A derivative, which is denoted as \(f'(x)\) or \(\frac{dy}{dx}\), represents the rate of change of a function with respect to a variable. Derivatives can be used to determine the slope of a function's graph at any point. For example, it can help us find local maxima and minima of functions by setting f'(x) = 0 and solving for x, which is known as critical point analysis. Here are some derivatives of common functions:
- For \(f(x) = x^n\), a power function, the derivative is \(f'(x) = nx^{n-1}\).
- For \(f(x) = \sin(x)\), a trigonometric function, the derivative is \(f'(x) = \cos(x)\).
- For \(f(x) = e^x\), an exponential function, the derivative is \(f'(x) = e^x\).
Chain Rule
The chain rule is a fundamental principle in calculus used to compute the derivative of a composite function. If \(y = f(u)\) and \(u = g(x)\), then the derivative of \(y\) with respect to \(x\) is given by \(\frac{dy}{dx} = \frac{dy}{du} \cdot \frac{du}{dx}\).
Continuity and Differentiability
Continuity
A function \(f(x)\) is continuous at a point \(a\) if \(\lim_{{x \to a}} f(x) = f(a)\). Continuity implies that there are no sudden jumps or breaks in the graph of the function at that point.
For instance, the function \(f(x) = x^2\) is continuous everywhere because \( \lim_{{x \to a}} x^2 = a^2 = f(a) \). On the other hand, a function like \(f(x) = \frac{1}{x}\) is not continuous at x = 0 because it has a vertical asymptote there, causing a break in the graph.
Differentiability
At any point a, a function can be said to be differentiable if its derivative can be represented as a single tangent line; differentiability requires no sharp corners or cusps at this location, and its rate of change remains consistent over time. An important benefit is its continuity; differentiable functions do not jump or break—for instance, the function \(f(x) = x^3\) is both continuous and differentiable everywhere it exists! However, not every continuous function may also be differentiated—for instance, the absolute value function \(f(x) = |x|\) at this location due to sharp corners at that location.
Basic Concepts of Integral Calculus
Integration
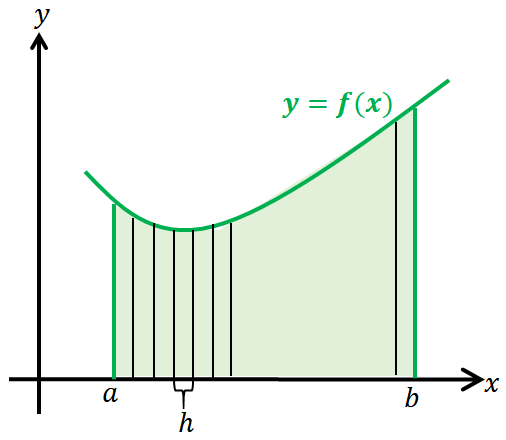
Integral calculus deals with accumulation and areas under curves; its key concepts are integration, definite integrals, and indefinite integrals. Integration refers to finding the integral of any function representing accumulation, either as quantities accumulated or areas beneath curves.
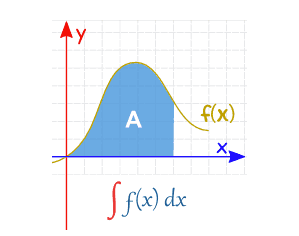
Definite Integral
A definite integral calculates the accumulation of a quantity over an interval \([a, b]\). It is denoted by \(\int_{a}^{b} f(x) \, dx\). Definite integrals have many uses in various areas, from calculating area under curves and total accumulation amounts to solving physical problems involving mass, charge, or probability distributions. Below are examples of definite integrals used with common functions:
- For \(f(x) = x\), \(\int_{a}^{b} x \, dx = \frac{b^2}{2} - \frac{a^2}{2}\).
- For \(f(x) = \sin(x)\), \(\int_{a}^{b} \sin(x) \, dx = -\cos(x) \bigg|_{a}^{b} = \cos(a) - \cos(b)\).
- For \(f(x) = e^x\), \(\int_{a}^{b} e^x \, dx = e^x \bigg|_{a}^{b} = e^b - e^a\).
Indefinite Integral
An indefinite integral represents the family of antiderivatives of a function, denoted by \(\int f(x) \, dx\), where the result is a general expression that includes a constant of integration (\( C \)). Indefinite integrals have an indispensable place in many mathematical and practical contexts, including solving differential equations, finding generalized solutions for accumulation problems, and modeling continuous growth/decay processes. Here are some examples of indefinite integrals for common functions:
- For \(f(x) = x^n\), \(\int x^n \, dx = \frac{x^{n+1}}{n+1} + C\).
- For \(f(x) = \cos(x)\), \(\int \cos(x) \, dx = \sin(x) + C\).
- For \(f(x) = e^x\), \(\int e^x \, dx = e^x + C\).
Other Topics in Calculus
Here we primarily highlight series, partial derivatives, and multiple integrals as examples—but for anyone wanting to go even deeper in learning calculus, we highly recommend Upstudy!
Partial Derivatives
Definition
Partial derivatives are derivatives of functions with multiple variables, such as f(x, y, z). They represent the rate of change of the function with respect to one variable while holding the other variables constant. For example, the partial derivative of f(x, y) with respect to x is denoted by \( \frac{\partial f}{\partial x} \), and it measures how f changes as x changes, keeping y fixed.
Chain Rule
The chain rule in multivariable calculus extends to partial derivatives. If \(z = f(x,y)\), then \(\frac{\partial z}{\partial t} = \frac{\partial z}{\partial x} \frac{\partial x}{\partial t} + \frac{\partial z}{\partial y} \frac{\partial y}{\partial t}\).
Multiple Integrals
Multiple integrals expand on integration to include functions with several variables and variables of multiple dimensions, enabling us to calculate quantities in three dimensions, such as surface area or mass of laminae. Triple integrals (\(\int \int \int f(x,y,z) \, dx \, dy \, dz\)) allow us to compute volumes in three-dimensional space—such as finding solid region volumes or total charges within electric fields, for example—providing precise analysis and calculations of spatial properties.
Infinite Series
Infinite series is a sum of infinitely many terms of a sequence, often written as \( \sum_{n=1}^{\infty} a_n \). Apart from infinite series, there are many other types of series, including powder series, which always be used by Mathematicians to represent functions, solve differential equations, and study convergence and divergence.
Common Theorems of Calculus
Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus creates a crucial link between differentiation and integration, consisting of two essential parts.
1. The first part states that if \( F \) is an antiderivative of \( f \) on the interval \([a, b]\), then the definite integral of \( f \) from \( a \) to \( b \) is given by \( \int_{a}^{b} f(x) \, dx = F(b) - F(a) \). This part establishes how the accumulation of a function’s values over an interval relates to its antiderivatives.
2. The second part asserts that if \( f \) is continuous on \([a, b]\), then the function \( F(x) = \int_{a}^{x} f(t) \, dt \) is continuous on \([a, b]\), differentiable on \((a, b)\), and its derivative is \( F'(x) = f(x) \). This connects the process of integration with differentiation, showing that integration can be reversed by differentiation.
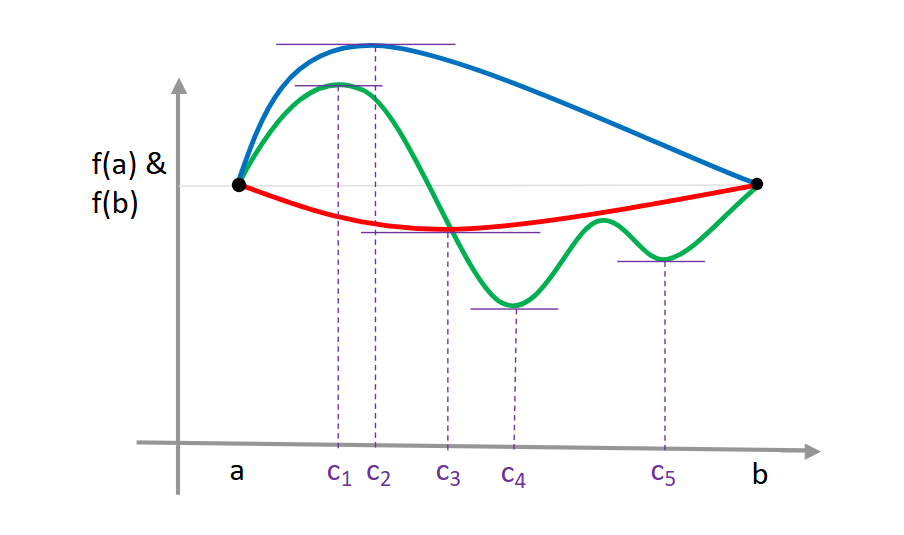
Rolle's Theorem
Rolle's Theorem is a fundamental result in calculus that provides conditions under which a function must have at least one point where its derivative is zero. It states that if a function \( f \) is continuous on a closed interval \([a, b]\), differentiable on the open interval \((a, b)\), and satisfies the condition \( f(a) = f(b) \), then there exists at least one point \( c \) within the open interval \((a, b)\) where the derivative \( f'(c) = 0 \). In other words, there is at least one point where the tangent to the curve is horizontal.
Taylor's Theorem
Taylor's Theorem provides an approximation of functions near points with polynomials. If a function f is infinitely differentiable at some point a, then an infinite sum of its derivatives at this point, known as a Taylor series, can be expressed to approximate it. The theorem is formally written as \( f(x) = \sum_{n=0}^{\infty} \frac{f^{(n)}(a)}{n!} (x - a)^n \), where \( f^{(n)}(a) \) represents the n-th derivative of f evaluated at a, and \( n! \) denotes the factorial of n.
Here are some examples:
1. Exponential Function (\( e^x \)):
\[e^x = \sum_{n=0}^{\infty} \frac{x^n}{n!} = 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \cdots\]
This series converges to \( e^x \) for all real \( x \).
2. Sine Function (\( \sin(x) \)):
\[\sin(x) = \sum_{n=0}^{\infty} \frac{(-1)^n}{(2n+1)!} x^{2n+1} = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots\]
This series converges to \( \sin(x) \) for all real \( x \).
3. Natural Logarithm (\( \ln(1+x) \)):
\[\ln(1+x) = \sum_{n=1}^{\infty} \frac{(-1)^{n+1}} {n} x^n = x - \frac{x^2}{2} + \frac{x^3}{3} - \frac{x^4}{4} + \cdots\]
This series converges for \( -1 \lt a x \le 1 \).
Applications of Calculus
Calculus' Role in Physics
Calculus plays an indispensable part in physical science. It allows scientists to describe and predict the behavior of physical systems by providing precise calculations that measure motion, forces, and energy; analyze electromagnetic fields; as well as understand thermodynamic principles. Calculus helps in many different applications within these disciplines, such as projectile trajectory using differential equations, force work performed across distance using integrals, and analyzing waveforms in electricity or magnetism, among many more uses.
Calculus in Economics
In economics, calculus plays an essential part in modeling and optimizing financial systems, identifying trends, predicting economic outcomes, and modeling consumer behavior analysis through utility maximization or demand functions that enable better prediction of market reactions or price changes. Differential calculus allows economists to calculate marginal costs/marginal revenues ratios, which allow them to assess marginal costs/marginal revenues ratios when producing one more unit of a product/service, while differential calculus allows economists to determine marginal costs/revenues ratios, which help economists analyze consumer behavior/market reactions/price changes more precisely by helping economic analysts to predict these numbers in advance.
