Who Invented Calculus? Newton vs. Leibniz & the History of Calculus
Explore the fascinating debate between Newton and Leibniz over the invention of calculus. Learn about its history, key concepts (limits, derivatives, integrals), and applications.
Isaac Turner
Dec 19, 2024 12 mins read
Does calculus exist, and who invented it? Inventor debate has long intrigued historians of mathematics; today calculus provides powerful tools that make modern physics, engineering, or economics manageable. Calculus' mathematical study of continuous change underlies many technological and scientific innovations we take for granted today and allows us to model phenomena ranging from planet motion and population growth through to market behavior in financial markets. Fortunately, calculus does exist, but its exact creator remains contentious among historians of mathematics—it remains controversial among some, and historians have long debated who invented calculus; among historians of mathematics, there seems to be controversy as historians discuss its origins, as there has been controversy for centuries, if any controversy.
Introduction to Calculus
Basic Definitions
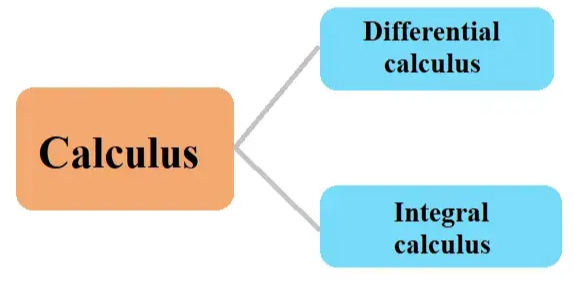
Calculus is an area of mathematics concerned with limits, functions, derivatives, integrals, and infinite series. It has two principal branches—differential calculus and integral calculus. Differential calculus deals with derivatives as rates of change, while integral calculus involves accumulation—while derivative calculus deals more specifically with differentials or derivatives of quantities, integral calculus deals instead with accumulation.
Key Concepts
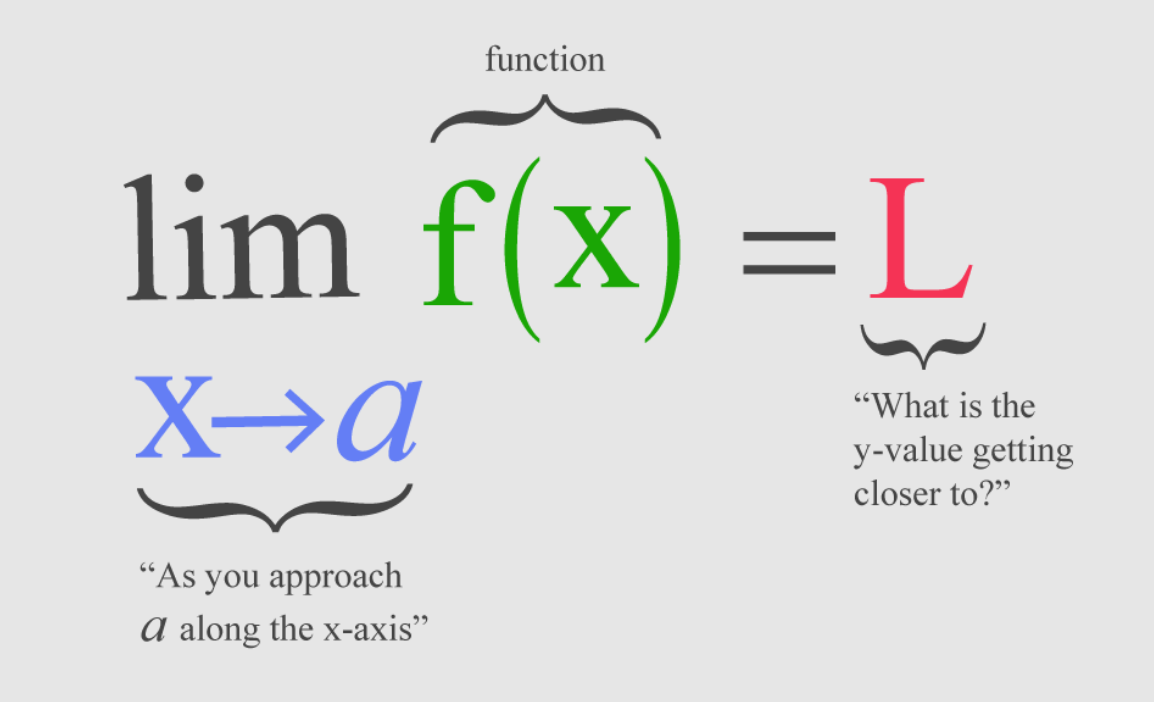
Limits
The concept of a limit is foundational in calculus and helps in understanding the behavior of functions as they approach specific points. A limit describes the value that a function approaches as the input (or variable) approaches some value. For instance, if we have a function \( f(x) \), the limit of \( f(x) \) as \( x \) approaches a particular value \( a \) is the value that \( f(x) \) gets closer to as \( x \) gets closer to \( a \). This is written as:
\[ \lim_{{x \to a}} f(x) = L \]
where \( L \) is the limit. Limits are crucial in defining both derivatives and integrals and are used to handle cases where functions do not behave nicely, such as when they have discontinuities or are not defined at certain points.
Derivatives
Derivatives measure how a function changes as its input changes. They represent the rate of change of a quantity. Formally, the derivative of a function \( f(x) \) at a point \( x = a \) is defined as the limit:
\[ f'(a) = \lim_{{h \to 0}} \frac{{f(a+h) - f(a)}}{h} \]
This expression gives the slope of the tangent line to the function at the point \( x = a \). Derivatives have widespread applications in various fields such as physics, engineering, and economics. For example, in physics, the derivative of the position of an object with respect to time is its velocity, and the derivative of velocity with respect to time is acceleration. Derivatives help in understanding and predicting how systems evolve over time.
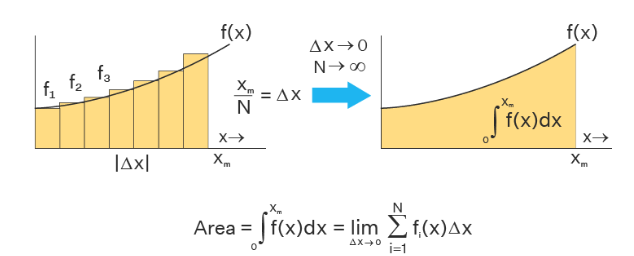
Integrals
Integrals are used to calculate the total accumulation of a quantity. The integral of a function over an interval gives the area under the curve of the function over that interval. The definite integral of a function \( f(x) \) from \( a \) to \( b \) is written as:
\[ \int_{a}^{b} f(x) \, dx \]
This represents the net area under the curve \( f(x) \) from \( x = a \) to \( x = b \). Integrals are used in numerous practical situations to calculate areas, volumes, and energy quantities in science. Integrals have their roots in calculus' Fundamental Theorem, which links integrals with derivatives so integration may be reversed by differentiation or vice versa.
You can also find problems applying these concepts in the UpStudy’s Study Bank.
Main Content
Primary Research Questions
Calculus explores how functions change and accumulate over time and space. Differential calculus examines rate-of-change questions by studying derivatives that track how quantities vary over time or space; integral calculus deals with accumulation by measuring areas under curves, total quantities and accumulation values using integrals; researchers may also investigate properties and behaviors of functions as well as convergence series or solutions to differential equations - this research forms the foundation for modeling real world problems encountered in fields like physics engineering economics etc.
Key Theorems
Differentiation Rules
Differentiation rules are essential tools for finding the derivative of a function. Some key rules include:
1. Power Rule: If \( f(x) = x^n \), then \( f'(x) = nx^{n-1} \).
2. Product Rule: If \( f(x) = u(x)v(x) \), then \( f'(x) = u'(x)v(x) + u(x)v'(x) \).
3. Quotient Rule: If \( f(x) = \frac{u(x)}{v(x)} \), then \( f'(x) = \frac{u'(x)v(x) - u(x)v'(x)}{[v(x)]^2} \).
4. Chain Rule: If \( f(x) = g(h(x)) \), then \( f'(x) = g'(h(x)) \cdot h'(x) \).
Chain rule calculations can help us easily and rapidly find the derivative of complex functions by breaking them into simpler parts and finding their derivative.
If you want to practice this, explore UpStudy’s derivatives calculator.
Mean Value Theorem
The Mean Value Theorem (MVT) is a fundamental result in calculus that provides a formal bridge between derivatives and integrals. There are several forms of the MVT, but two of the most important are:
Lagrange's Mean Value Theorem
Lagrange's MVT states that for a continuous function \( f \) on the closed interval \([a, b]\) and differentiable on the open interval \((a, b)\), there exists at least one point \( c \in (a, b) \) where:
\[ f'(c) = \frac{f(b) - f(a)}{b - a} \]
This means that there is at least one point where the instantaneous rate of change (the derivative) equals the average rate of change over the interval.
Cauchy's Mean Value Theorem
Cauchy's MVT generalizes Lagrange's MVT. For two functions \( f \) and \( g \) that are continuous on \([a, b]\) and differentiable on \((a, b)\), there exists at least one point \( c \in (a, b) \) where:
\[ \frac{f'(c)}{g'(c)} = \frac{f(b) - f(a)}{g(b) - g(a)} \]
This theorem is useful in various applications, including proving L'Hôpital's rule.
Both of theorems play an indispensable part in comprehending how functions behave, playing an essential part in mathematical analysis and its applications.
Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is an iconic statement in calculus which highlights that differentiation and integration are fundamentally opposite processes.The theorem has two main parts:
1. First Part: This part states that if \( f \) is a continuous function on the interval \([a, b]\) and \( F \) is an antiderivative of \( f \) on \([a, b]\), then:
\[ \int_{a}^{b} f(x) \, dx = F(b) - F(a) \]
This means that the definite integral of \( f \) from \( a \) to \( b \) is equal to the difference in the values of its antiderivative at the endpoints of the interval.
2. Second Part: This part states that if \( F \) is defined by:
\[ F(x) = \int_{a}^{x} f(t) \, dt \]
for \( x \) in \([a, b]\), and \( f \) is continuous, then \( F \) is differentiable on \((a, b)\), and its derivative is \( f \):
\[ F'(x) = f(x) \]
This means that the derivative of the integral function \( F \) is the original function \( f \).
Together, these parts form an inextricable link between differentiation and integration, significantly simplifying computation of definite integrals while offering powerful tools for solving an array of mathematical and applied sciences problems.
"If you are interested in its proof process, you can visit UpStudy's Ask Tutors to consult our live tutors."
Historical Background of Calculus Creation
Ancient Precursors
Ancient Egypt
Mathematicians in ancient Egypt used simple forms of calculus to solve area and volume-related problems. Egyptian mathematicians devised methods of approximating both circular area and volume estimation. Their efforts laid the groundwork for later developments of integral calculus.
Ancient Greece
Greek mathematicians such as Eudoxus and Archimedes made significant contributions to the early development of calculus. Eudoxus advanced exhaustion theory - an precursor of limit theory - for finding areas and volumes, while Archimedes used exhaustion analysis to calculate both areas under parabolas as well as volumes for spheres using exhaustion's method - becoming one of antiquity's greatest mathematicians.
China
Liu Hui and Zu Chongzhi of ancient China made important strides that presaged calculus. Liu used an approach similar to exhaustion when calculating circle areas while Zu approximated pi's value with remarkable precision - both contributions demonstrating global aspects of early mathematical development.
Middle Ages
Middle East
Middle Age Islamic mathematicians preserved and expanded upon the knowledge of Ancient Greece through Islamic mathematicians like Al-Khwarizmi, Omar Khayyam, Alhazen's studies in optics, light studies and Alhazen. Scholars such as these made significant contributions in algebra, geometry and calculus that later inspired its development - eventually giving way to calculus itself! Alhazen's studies in optics laid a solid basis for modern mathematical physics research.
India
Indian mathematicians made significant contributions to the development of calculus. Aryabhata and Bhaskara II worked on problems concerning infinitesimals while creating early forms of differential calculus, while Madhava from Sangamagrama in Kerala developed series expansions for trigonometric functions - work which anticipated later European mathematical developments.
The Controversy
Invention Process
Newton's Invention Process
Isaac Newton first devised his version of calculus, known as "the method of fluxions," during the 1660s. Newton's work on calculus was deeply intertwined with his investigation of motion and change; particularly as applied to planet orbits or physical bodies' behavior. Newton popularized the term "fluxion," or the instantaneous rate of change of any quantity instantaneously over time; today we understand this term to represent derivatives.
Additionally, Newton came up with another idea called an "inverse fluxion," similar to our understanding of an integral. He laid the groundwork for classical mechanics by employing these concepts to formulate laws of motion and universal gravitation. While Newton did not immediately publish his findings, his notebooks and manuscripts from this period show early developments of calculus which helped solve issues in both physics and astronomy.
Leibniz's Invention Process
Gottfried Wilhelm Leibniz, a German mathematician and philosopher, independently developed calculus in the late 1670s. His work was distinct from Isaac Newton's, both in approach and notation. Leibniz's version of calculus, known as "calculus differentialis" and "calculus integralis," introduced the integral and differential symbols (\(\int\) and \(d\)), which are still in use today.
Leibniz adopted an approach which was more systematic and formalized compared to that taken by Newton. He focused on manipulating infinitesimals, leading him to develop more intuitive rules of differentiation and integration that were simpler for mathematicians to apply. Leibniz's notation and methods had an enormous effect on future developments of calculus as it made calculus more readily accessible and approachable by mathematicians alike.
Leibniz laid the groundwork for formal development of calculus as a mathematical discipline, although his contributions remain controversial today. Modern historians acknowledge his significance to mathematics' development; even today his notation remains standard across fields like calculus.
Emergence of Conflict
Inception
Isaac Newton and Gottfried Wilhelm Leibniz began clashing over the invention of calculus in late 17th century England. While both mathematicians independently created calculus independently but with different methodologies and notations, controversy began between the two when Newton accused Leibniz of plagiarizing unpublished work after Leibniz published findings which seemed similar. Nationalistic sentiments further complicated matters between English mathematicians supporting Newton against Continental Europeans supporting Leibniz; The Royal Society led by Newton published a report accusing Leibniz of intellectual theft which hindered collaboration among mathematicians slowing progress of mathematical research progress significantly.
Respective Evidence
Newton's Evidence
Isaac Newton created his version of calculus, known as the "method of fluxions," during the mid-1660s. To support his claim, Newton produced early manuscripts and notebooks outlining his ideas about calculus; these documents date from 1660s were filled with detailed accounts on finding tangents and areas essential aspects of differential and integral calculus; to illustrate his claim his supporters pointed to his early unpublished works as proof that Newton invented calculus first while correspondence from other mathematicians such as John Wallis also provided proof.
Leibniz's Evidence
Gottfried Wilhelm Leibniz independently developed his version of calculus between 1677 and 1680 independently. Leibniz provided evidence for this claim through published papers such as his 1684 paper entitled, "Nova Methodus pro Maximis et Minimis." Here he introduced differential calculus; later that year (1686) Leibniz released another paper which elaborated upon integral calculus further. Leibniz's notation, including the symbols \( \int \) for integrals and \( d \) for differentials, became widely adopted and remain in use today. Leibniz's supporters highlighted his systematic, formalized approach to calculus. Their claims rested on Leibniz's published works providing clear methods of performing calculus operations.
Both Newton and Leibniz offered compelling evidence supporting their claims of independent discovery of calculus, leading to an extended and contentious debate as to who exactly invented calculus.
Newton's Attacks on Leibniz
Conflict escalated when Newton accused Leibniz of plagiarism. Newton led his Royal Society of London, publishing in 1712 what became known as the "Commercium Epistolicum", accusing Leibniz of plagiarizing ideas from unpublished manuscripts of Newton that Leibniz claimed were stolen from. Leibniz responded that his concepts had evolved independently despite both sides engaging in personal attacks and public debates over this dispute. The dispute eventually turned extremely nasty between them all involved, becoming personal attacks between individual scientists as well as public debates among experts among scientists across disciplines - until all involved reached consensus among scholars on various subjects in 1712.
Conclusions
Contemporary Result
Throughout their lives, disagreement over who invented calculus was left unresolved. Both Newton and Leibniz continued developing different versions of it while their followers split over which method would prevail. Such disagreement hampered collaboration and communication among mathematicians from different countries and ultimately hindered mathematical research progress.
Modern Conclusions
Modern historians of mathematics generally acknowledge Newton and Leibniz independently created calculus, though their approaches and notations often diverged significantly. Today, calculus is widely recognized as having been co-created; both men are honored for their pioneering contributions.
Global Recognition of Newton and Leibniz
Distinct Characteristics of Newton's and Leibniz's Theories
Newton's calculus was more geometric in nature and focused around fluxions to describe changes to quantities; while Leibniz's focused more analytically on manipulating infinitesimals. Their differing styles had profound influences over subsequent mathematics branches as well as applications.
Evaluation of Their Contributions
Both Newton and Leibniz made significant contributions to calculus and mathematics as a whole, including Newton laying the groundwork for classical mechanics and motion studies while Leibniz provided notation and formalalism tools to further mathematical development. Their combined work had profound ramifications on science, engineering and technology development.
Significance of Calculus
Significance in Mathematical Theory
Calculus has transformed mathematical theory by offering an framework to understand continuous change and allow mathematicians to create new theories or solve intractable problems more easily than before. Calculus concepts like limits, derivatives and integrals form the core of many areas of mathematics including analysis, differential equations and topology as well as having significant influences over other branches such as complex analysis or functional analysis.
Applications in Other Fields
Physics
Calculus is essential in physical science for modeling and understanding physical systems, from modeling motion, forces, energy flows to solving differential equations that govern physical phenomena such as electromagnetism, thermodynamics, quantum mechanics or general relativity. It plays an integral part of studies such as electromagnetism thermodynamics quantum mechanics general relativity among others.
Medicine
Calculus can play an invaluable role in medicine, particularly the modeling and analysis of biological systems and medical data. Calculus has long been employed as a powerful way of simulating biological systems such as diseases spreading or populations growing exponentially over time; and simulating physiological systems' behavior such as those used by MRI/CT scans in reconstructing images from raw data sets.
Economics
Calculus is used extensively in economics for modeling and analyzing dynamic systems like market behavior or economic expansion, marginal costs and revenues calculation, optimizing production/consumption scheduling and tracking agents over time as well as understanding their behavioral changes over time. Calculus also plays a central role in econometrics to analyze/interpret economic data sets.